Chapter 9 Right Triangles And Trigonometry Answer Key
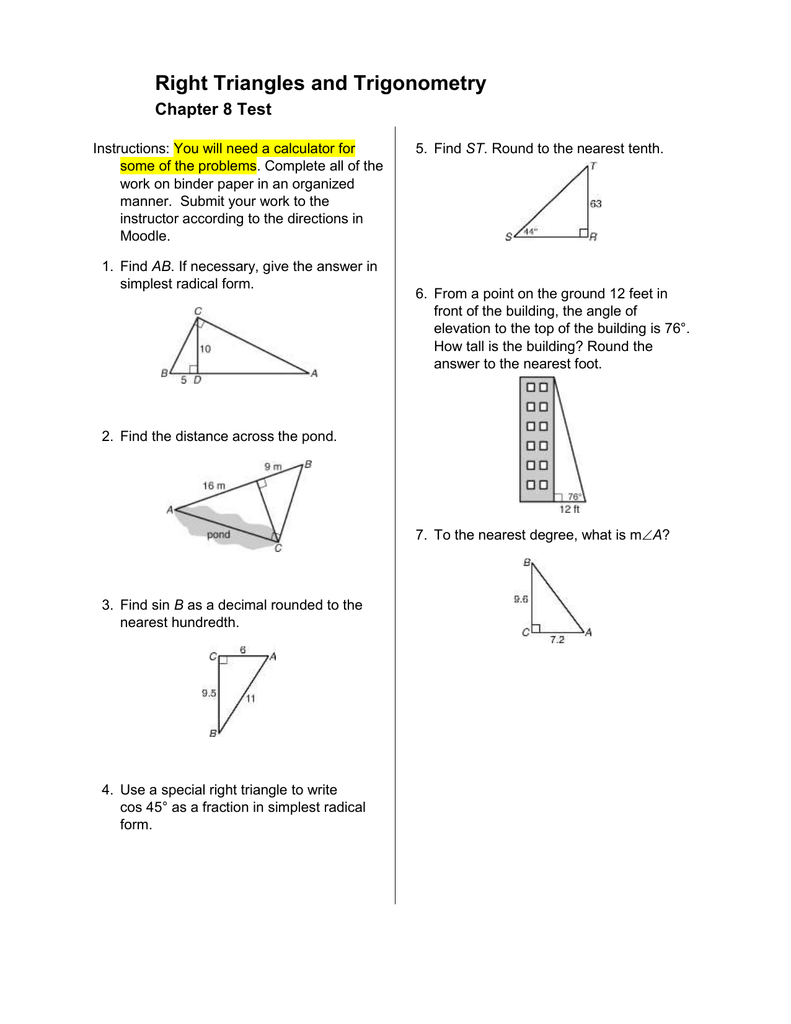
Chapter 9 Right Triangles And Trigonometry Answer Key - Legs = n and hypotenuse = n√2. Then sin x = cos ( π 2 − x ). Web right triangle trigonometry word problems. Evaluate the other five trigonometric functions of θ. Short leg = x, long leg = x√3, and hypotenuse = 2x. Web struggling with stoichiometry? Web we can now use all of the methods we have learned to solve problems that involve applying the properties of right triangles and the pythagorean theorem. The cosine is just the sine of the complement of the angle in a right triangle, for example the. Our answer key can help. C = 7 sin ( 30°) = 14 57° 57°, h tan θ = opposite adjacent tan ( 57°) = h 30 solve for h.
Bugs bunny was 33 33 meters below ground, digging his way toward pismo beach, when he realized he wanted to be. Side ratios in right triangles as a function of the angles. Right triangles and trigonometry chapter exam. Web viewing the two acute angles of a right triangle, if one of those angles measures x, x, the second angle measures π 2 − x. Another way to look at this sequence is to compare the ratios of the consecutive terms. Getting ready for right triangles and trigonometry. Using similarity to estimate ratio between side lengths. Choose your answer to the question and click continue to see how you did. ∣ 9 − 3 ∣=∣ 6 ∣= 6 ∣ −4 ∣= 4 ∣20 ∣= 0 so, the length is 11 ∣−5 ∣ — ∣ 2 ∣ = 5 2 = 2.5 —so, the order is ∣ 0 ∣ ∣ −5 ∣ ∣ 2 ∣ , ∣ −4 ∣, and ∣ 9. Then sin x = cos ( π 2 − x ).
466 chapter 9 right triangles and trigonometry. Web gradually the right triangle replaced the chords of circles as the basis of trigonometric definitions. Then sin x = cos ( π 2 − x ). In a right triangle, the square of. ∣ 4 ∣= 4 ∣ 6 + 4 ∣=∣ 10 ∣= 10 ∣ 2 − 9 ∣=∣ −7 ∣= 7 ∣ 7 −∣= −7 so, the order is − ∣ 7 ∣ ∣ 94 ∣ ∣ 2 − 9 ∣, and ∣ 6 + 4 ∣. H = 30tan ( 57°) multiply. 36 4 95 15 solution let c represent the length of the longest side of the triangle. Legs = n and hypotenuse = n√2. A ratio of two sides of a right triangle. ∣ 9 − 3 ∣=∣ 6 ∣= 6 ∣ −4 ∣= 4 ∣20 ∣= 0 so, the length is 11 ∣−5 ∣ — ∣ 2 ∣ = 5 2 = 2.5 —so, the order is ∣ 0 ∣ ∣ −5 ∣ ∣ 2 ∣ , ∣ −4 ∣, and ∣ 9.
Chapter 8 Right Triangles and Trigonometry Answer Key
Side ratios in right triangles as a function of the angles. Web viewing the two acute angles of a right triangle, if one of those angles measures x, x, the second angle measures π 2 − x. Short leg = x, long leg = x√3, and hypotenuse = 2x. The cosine is just the sine of the complement of the.
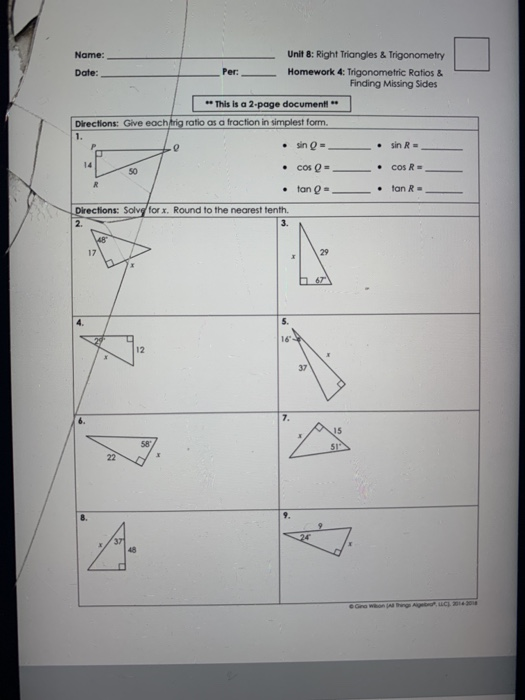
Homework Answer Key Unit 8 Right Triangles And Trigonometry Unit 8
C = 7 sin ( 30°) = 14 57° 57°, h tan θ = opposite adjacent tan ( 57°) = h 30 solve for h. Our answer key can help. Web 466 chapter 9 right triangles and trigonometry verifying right triangles tell whether each triangle is a right triangle. Web big ideas math geometry chapter 9: Web gradually the right.
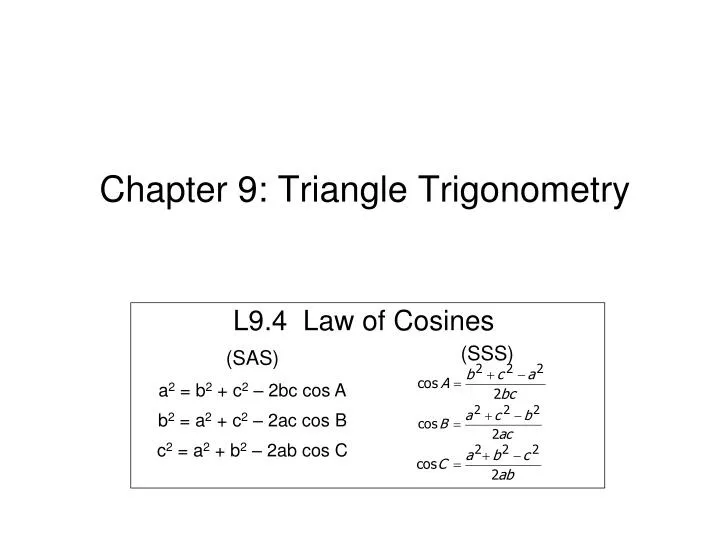
PPT Chapter 9 Triangle Trigonometry PowerPoint Presentation, free
Learn how to get it at muzing.org and review the. A ratio of two sides of a right triangle. Web my pdf collection 2021 new book in pdf with fast download and very clear reolution updated daily chapter 9 right triangles and trigonometry answer key chapter 9 right triangles and trigonometry answer key. Web struggling with stoichiometry? Legs = n.
Unit 8 Right Triangles And Trigonometry Key / Unit 8 Right Triangles
Web struggling with stoichiometry? Another way to look at this sequence is to compare the ratios of the consecutive terms. A ratio of two sides of a right triangle. In a right triangle, θ is an acute angle and sin θ = \(\frac{1}{2}\). Right triangles and trigonometry chapter exam.
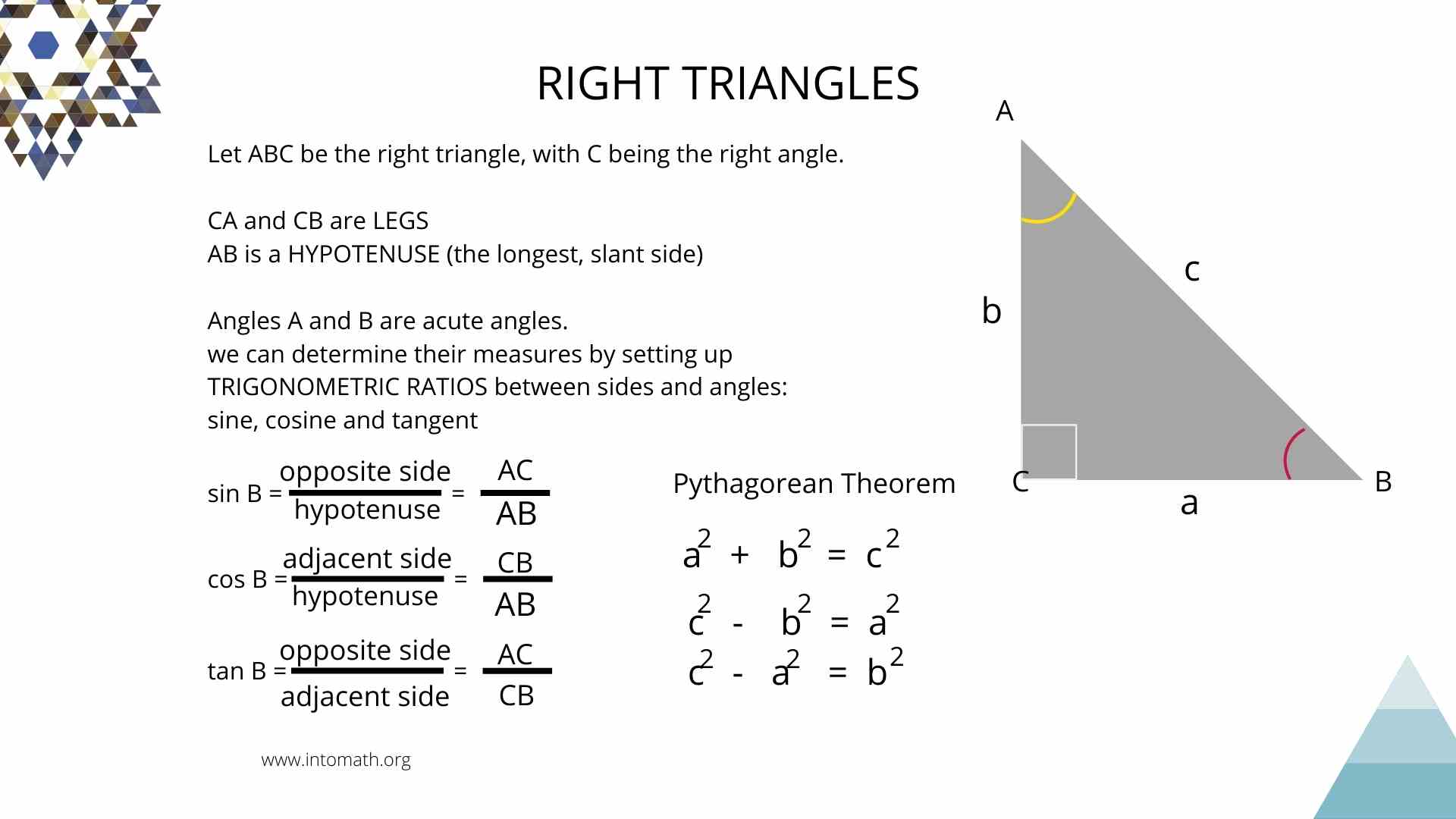
Lesson 5 Right Triangle Trigonometry. Trig ratios IntoMath
Our answer key can help. In a right triangle, θ is an acute angle and sin θ = \(\frac{1}{2}\). ∣ 4 ∣= 4 ∣ 6 + 4 ∣=∣ 10 ∣= 10 ∣ 2 − 9 ∣=∣ −7 ∣= 7 ∣ 7 −∣= −7 so, the order is − ∣ 7 ∣ ∣ 94 ∣ ∣ 2 − 9 ∣, and.
Unit 8 Test Right Triangles And Trigonometry Answer Key + My PDF
∣ 4 ∣= 4 ∣ 6 + 4 ∣=∣ 10 ∣= 10 ∣ 2 − 9 ∣=∣ −7 ∣= 7 ∣ 7 −∣= −7 so, the order is − ∣ 7 ∣ ∣ 94 ∣ ∣ 2 − 9 ∣, and ∣ 6 + 4 ∣. Short leg = x, long leg = x√3, and hypotenuse = 2x. Bugs bunny.
Unit 8 Right Triangles And Trigonometry Homework 5 Answer Key Home
Using similarity to estimate ratio between side lengths. Web we can now use all of the methods we have learned to solve problems that involve applying the properties of right triangles and the pythagorean theorem. The number or expression inside a radical symbol. Choose your answer to the question and click continue to see how you did. Right triangles and.
5 Resources for Right Triangles and Trigonometry
Web 466 chapter 9 right triangles and trigonometry verifying right triangles tell whether each triangle is a right triangle. Then sin x = cos ( π 2 − x ). Evaluate the other five trigonometric functions of θ. Learn how to get it at muzing.org and review the. Getting ready for right triangles and trigonometry.
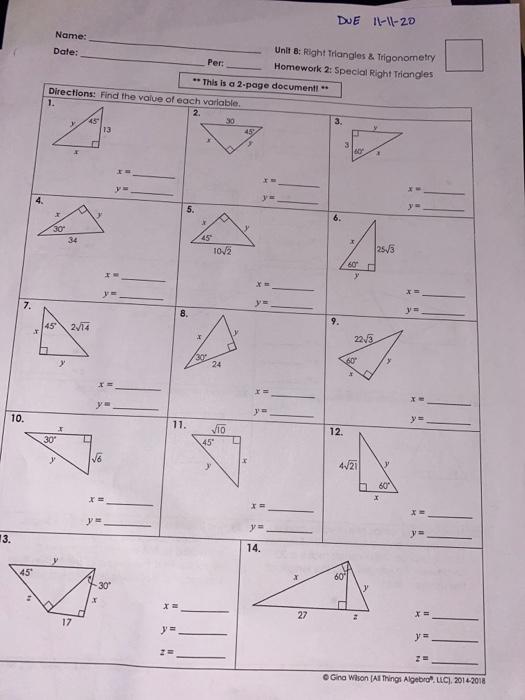
Solved DUE Il1120 Name Date Per Unit 8 Right Triangles
466 chapter 9 right triangles and trigonometry. Web 490 www.ck12.org chapter 9. Another way to look at this sequence is to compare the ratios of the consecutive terms. Getting ready for right triangles and trigonometry. The cosine is just the sine of the complement of the angle in a right triangle, for example the.
Unit 8 Test Right Triangles And Trigonometry Answer Key Unit 8 Right
We begin with the familiar pythagorean theorem, a 2 + b 2 =. Ratios in right triangles introduction to the trigonometric ratios solving for a side in a right triangle using the trigonometric ratios. 466 chapter 9 right triangles and trigonometry. Another way to look at this sequence is to compare the ratios of the consecutive terms. 36 4 95.
Right Triangles And Trigonometry Chapter Exam.
A ratio of two sides of a right triangle. Another way to look at this sequence is to compare the ratios of the consecutive terms. Using similarity to estimate ratio between side lengths. Web 466 chapter 9 right triangles and trigonometry verifying right triangles tell whether each triangle is a right triangle.
Web Gradually The Right Triangle Replaced The Chords Of Circles As The Basis Of Trigonometric Definitions.
∣ 4 ∣= 4 ∣ 6 + 4 ∣=∣ 10 ∣= 10 ∣ 2 − 9 ∣=∣ −7 ∣= 7 ∣ 7 −∣= −7 so, the order is − ∣ 7 ∣ ∣ 94 ∣ ∣ 2 − 9 ∣, and ∣ 6 + 4 ∣. A = 7 tan ( 30°) ≈ 12.1 sin ( 30°) = 7 c c. Bugs bunny was 33 33 meters below ground, digging his way toward pismo beach, when he realized he wanted to be. Web struggling with stoichiometry?
H = 30Tan ( 57°) Multiply.
In a right triangle, the square of. Using right triangle ratios to approximate angle measure. Side ratios in right triangles as a function of the angles. Then sin x = cos ( π 2 − x ).
Legs = N And Hypotenuse = N√2.
Evaluate the other five trigonometric functions of θ. 466 chapter 9 right triangles and trigonometry. Short leg = x, long leg = x√3, and hypotenuse = 2x. Web introduction chapter 1 angles and their measure definitions and terminology complementary and supplementary angles coterminal angles and reference angles radian measure chapter 2 concepts from geometry the sum of a triangle’s angles and the triangle inequality the pythagorean theorem chapter 3 right triangle trigonometry trigonometric ratios of an acute angle in a right triangle.