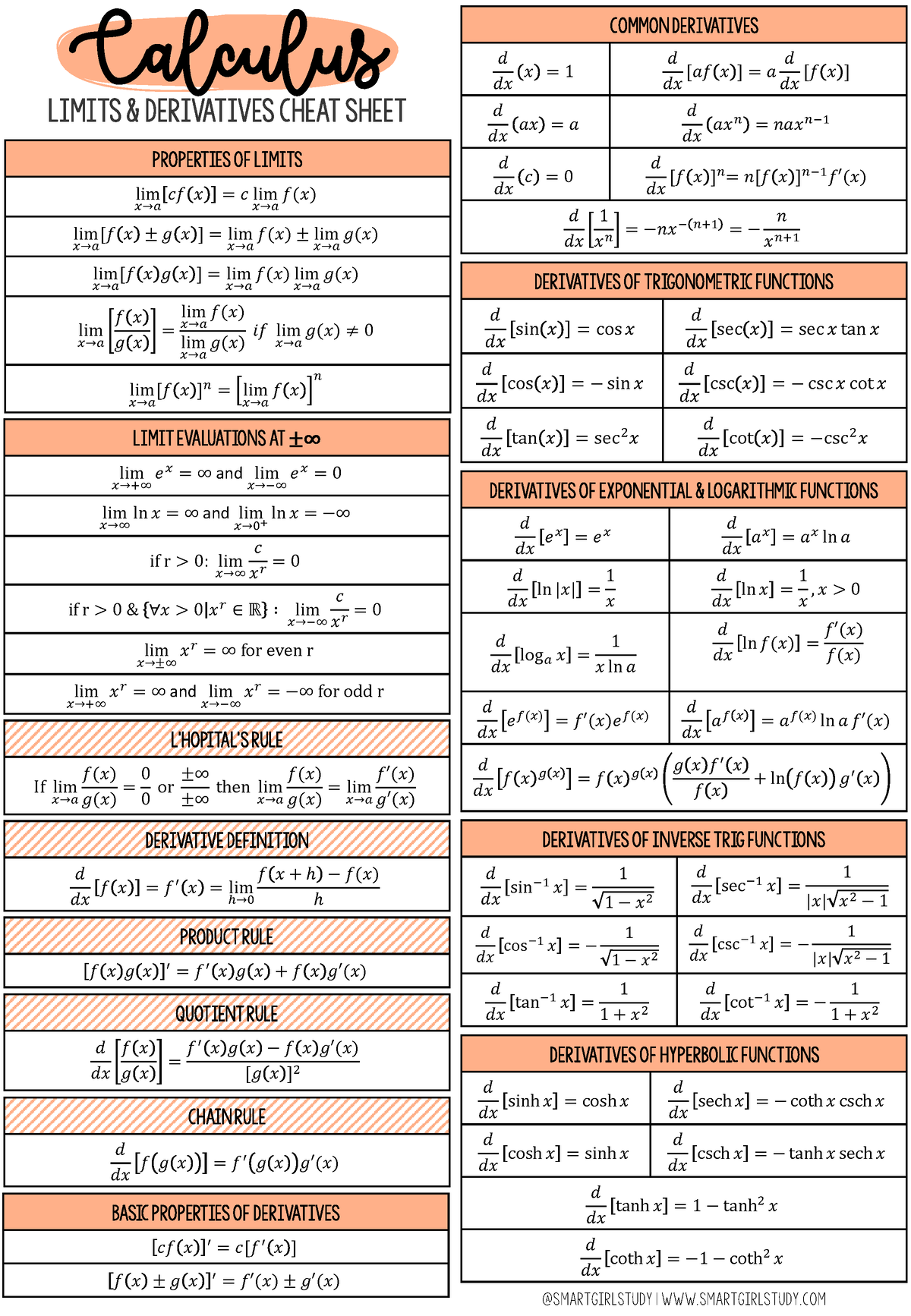
Limits Cheat Sheet

Limits Cheat Sheet - Ds = 1 dy ) 2. Where ds is dependent upon the form of the function being worked with as follows. 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Same definition as the limit except it requires x. Lim 𝑥→ = • squeeze theorem: Let , and ℎ be functions such that for all ∈[ , ]. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. • limit of a constant: Lim 𝑥→ = • basic limit:

Let , and ℎ be functions such that for all ∈[ , ]. 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. • limit of a constant: Lim 𝑥→ = • squeeze theorem: Same definition as the limit except it requires x. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. Where ds is dependent upon the form of the function being worked with as follows. Ds = 1 dy ) 2. Lim 𝑥→ = • basic limit:

Lim 𝑥→ = • basic limit: 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Ds = 1 dy ) 2. Lim 𝑥→ = • squeeze theorem: Let , and ℎ be functions such that for all ∈[ , ]. Where ds is dependent upon the form of the function being worked with as follows. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. • limit of a constant: Same definition as the limit except it requires x.

Calculus Limits Cheat Sheet

2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. Where ds is dependent upon the form of the function being.

Indeterminate forms of limits Math Worksheets & Math Videos Ottawa

Lim 𝑥→ = • basic limit: Where ds is dependent upon the form of the function being worked with as follows. Ds = 1 dy ) 2. 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Same definition as the limit except it requires x.

Civil Law Time Limits Cheat Sheet Noah F. Schwinghamer, Esq

Same definition as the limit except it requires x. Where ds is dependent upon the form of the function being worked with as follows. • limit of a constant: Ds = 1 dy ) 2. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without.

SOLUTION Limits cheat sheet Studypool

Lim 𝑥→ = • squeeze theorem: 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Where ds is dependent upon the form of the function being worked with as follows. Ds = 1 dy ) 2. Web we can make f(x) as close to l as we want by.

Calculus Limits Cheat Sheet Calculus, Rational expressions, Precalculus

• limit of a constant: Ds = 1 dy ) 2. 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Where ds is dependent upon the form of the function being worked with as follows. Let , and ℎ be functions such that for all ∈[ , ].

Limits Worksheet With Answers Worksheet Now

Lim 𝑥→ = • basic limit: Let , and ℎ be functions such that for all ∈[ , ]. Where ds is dependent upon the form of the function being worked with as follows. Same definition as the limit except it requires x. Web we can make f(x) as close to l as we want by taking x sufficiently close.

Limits Calculus Cheat Sheet Calculus Cheat Sheet

Where ds is dependent upon the form of the function being worked with as follows. • limit of a constant: Lim 𝑥→ = • squeeze theorem: 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Let , and ℎ be functions such that for all ∈[ , ].

Calculus Cheat Sheet i dont know la Limits & Derivatives Cheat

Ds = 1 dy ) 2. Let , and ℎ be functions such that for all ∈[ , ]. Lim 𝑥→ = • squeeze theorem: Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. 2 dy y = f (.

Pin on Math cheat sheet

Ds = 1 dy ) 2. Where ds is dependent upon the form of the function being worked with as follows. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. Same definition as the limit except it requires x. Let.

Calculus Cheat Sheet All Limits Definitions Precise Definition We

Where ds is dependent upon the form of the function being worked with as follows. 2 dy y = f ( x ) , a £ x £ b ds = ( dx ) +. Same definition as the limit except it requires x. Let , and ℎ be functions such that for all ∈[ , ]. Web we can.

Same Definition As The Limit Except It Requires X.

Where ds is dependent upon the form of the function being worked with as follows. Web we can make f(x) as close to l as we want by taking x sufficiently close to a (on either side of a) without letting x = a. Ds = 1 dy ) 2. • limit of a constant: